Abstract
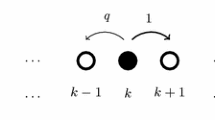
A simple asymmetric exclusion model with open boundaries is solved exactly in one dimension. The exact solution is obtained by deriving a recursion relation for the steady state: if the steady state is known for all system sizes less thanN, then our equation (8) gives the steady state for sizeN. Using this recursion, we obtain closed expressions (48) for the average occupations of all sites. The results are compared to the predictions of a mean field theory. In particular, for infinitely large systems, the effect of the boundary decays as the distance to the power −1/2 instead of the inverse of the distance, as predicted by the mean field theory.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
T. M. Liggett,Interacting Particle Systems (Springer-Verlag, New York, 1985).
R. Kutner,Phys. Lett. A 81:239 (1981).
H. van Beijeren, K. W. Kehr, and R. Kutner,Phys. Rev. B 28:5711 (1983).
P. A. Ferrari,Ann. Prob. 14:1277 (1986).
A. De Masi and P. Ferrari,J. Stat. Phys. 36:81 (1984).
D. Kandel and E. Domany,J. Stat. Phys. 58:685 (1990); D. Kandel, E. Domany, and B. Nienhuis,J. Phys. A 23:L755 (1990).
J. P. Marchand and P. A. Martin,J. Stat. Phys. 44:491 (1986).
D. Dhar,Phase Transitions 9:51 (1987).
J. Krug and H. Spohn, inSolids far from Equilibrium: Growth, Morphology and Defects, C. Godreche, ed. (Cambridge University Press, Cambridge, 1991).
M. Kardar, G. Parisi, and Y. Zhang,Phys. Rev. Lett. 56:889 (1986).
J. Krug,Phys. Rev. Lett. 67:1882 (1991).
D. E. Wolf and L. H. Tang,Phys. Rev. Lett. 65:1591 (1990).
D. Kandel and D. Mukamel,Europhys. Lett. (1992), in press.
J. Cook and D. E. Wolf,J. Phys. A 24:L351 (1991).
H. Rost,Z. Wahrsch. Verw. Geb. 58:41 (1981).
A. Galves, C. Kipnis, C. Macchioro, and E. Presutti,Commun. Math. Phys. 81:127 (1981).
B. Derrida, J. L. Lebowitz, E. R. Speer, and H. Spohn,Phys. Rev. Lett. 67:165 (1991);J. Phys. A 24:4805 (1991).
S. A. Janowsky and J. L. Lebowitz,Phys. Rev. A 45:618 (1992).
L. H. Gwa and H. Spohn,Phys. Rev. Lett. 68:725 (1992).
H. van Beijeren, R. Kutner, and H. Spohn,Phys. Rev. Lett. 54:2026 (1985).
J. Krug, Private communication.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Derrida, B., Domany, E. & Mukamel, D. An exact solution of a one-dimensional asymmetric exclusion model with open boundaries. J Stat Phys 69, 667–687 (1992). https://doi.org/10.1007/BF01050430
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01050430