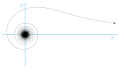
Спираль
Спираль — хăшпĕр йышши йĕрсем тĕлĕшпе историлле майпа çирĕпленне ят.
Çав вăхăтрах спираль пирки математика енчен тĕллĕхлĕ палăртавсем те майлаштараççĕ.
Лаптак спиральсем
[тӳрлет | кодне тӳрлет]-
Архимед спиралĕ
-
Корню спиралĕ
-
Ферма спиралĕ
-
Гиперболăлла спираль
-
lituus
-
Логарифмла спираль
-
Феодор спиралĕ
-
Фибоначчи спиралĕ (Ылттăн спираль)
-
Çаврашка инволюти (хури) Архимед спиралĕпе (хĕрли) пĕр мар
Уçлăхри спиральсем
[тӳрлет | кодне тӳрлет]Сферăлла спираль
[тӳрлет | кодне тӳрлет]Çавăн пекех
[тӳрлет | кодне тӳрлет]Асăрхавсем
[тӳрлет | кодне тӳрлет]Литература
[тӳрлет | кодне тӳрлет]- Cook, T., 1903. Spirals in nature and art. Nature 68 (1761), 296.
- Cook, T., 1979. The curves of life. Dover, New York.
- Habib, Z., Sakai, M., 2005. Spiral transition curves and their applications. Scientiae Mathematicae Japonicae 61 (2), 195—206.
- Dimulyo, S., Habib, Z., Sakai, M., 2009. Fair cubic transition between two circles with one circle inside or tangent to the other. Numerical Algorithms 51, 461—476 [1] 2018 ҫулхи Чӳк уйӑхӗн 27-мӗшӗнче архивланӑ.Шаблон:Недоступная ссылка.
- Harary, G., Tal, A., 2011. The natural 3D spiral. Computer Graphics Forum 30 (2), 237—246 [2] 2015 ҫулхи Чӳк уйӑхӗн 22-мӗшӗнче архивланӑ..
- Xu, L., Mould, D., 2009. Magnetic curves: curvature-controlled aesthetic curves using magnetic fields. In: Deussen, O., Hall, P. (Eds.), Computational Aesthetics in Graphics, Visualization, and Imaging. The Eurographics Association [3].
- Wang, Y., Zhao, B., Zhang, L., Xu, J., Wang, K., Wang, S., 2004. Designing fair curves using monotone curvature pieces. Computer Aided Geometric Design 21 (5), 515—527 [4].
- A. Kurnosenko. Applying inversion to construct planar, rational spirals that satisfy two-point G2 Hermite data. Computer Aided Geometric Design, 27(3), 262—280, 2010 [5].
- A. Kurnosenko. Two-point G2 Hermite interpolation with spirals by inversion of hyperbola. Computer Aided Geometric Design, 27(6), 474—481, 2010.
- Miura, K.T., 2006. A general equation of aesthetic curves and its self-affinity. Computer-Aided Design and Applications 3 (1-4), 457—464 [6].
- Miura, K., Sone, J., Yamashita, A., Kaneko, T., 2005. Derivation of a general formula of aesthetic curves. In: 8th International Conference on Humans and Computers (HC2005). Aizu-Wakamutsu, Japan, pp. 166—171 [7].
- Meek, D., Walton, D., 1989. The use of Cornu spirals in drawing planar curves of controlled curvature. Journal of Computational and Applied Mathematics 25 (1), 69-78 [8].
- Farin, G., 2006. Class A Bézier curves. Computer Aided Geometric Design 23 (7), 573—581 [9].
- Farouki, R.T., 1997. Pythagorean-hodograph quintic transition curves of monotone curvature. Computer-Aided Design 29 (9), 601—606.
- Yoshida, N., Saito, T., 2006. Interactive aesthetic curve segments. The Visual Computer 22 (9), 896—905 [10] 2016 ҫулхи Пуш уйӑхӗн 4-мӗшӗнче архивланӑ..
- Yoshida, N., Saito, T., 2007. Quasi-aesthetic curves in rational cubic Bézier forms. Computer-Aided Design and Applications 4 (9-10), 477—486 [11] 2016 ҫулхи Пуш уйӑхӗн 3-мӗшӗнче архивланӑ..
- Ziatdinov, R., Yoshida, N., Kim, T., 2012. Analytic parametric equations of log-aesthetic curves in terms of incomplete gamma functions. Computer Aided Geometric Design 29 (2), 129—140 [12].
- Ziatdinov, R., Yoshida, N., Kim, T., 2012. Fitting G2 multispiral transition curve joining two straight lines, Computer-Aided Design 44(6), 591—596 [13].
- Ziatdinov, R., 2012. Family of superspirals with completely monotonic curvature given in terms of Gauss hypergeometric function. Computer Aided Geometric Design 29(7): 510—518, 2012 [14].
- Ziatdinov, R., Miura K.T., 2012. On the Variety of Planar Spirals and Their Applications in Computer Aided Design. European Researcher 27(8-2), 1227—1232 [15] 2020 ҫулхи Утӑ уйӑхӗн 23-мӗшӗнче архивланӑ..
Йĕрсем |
|
|---|---|
| Палăртавсем | |
| Улшăвланисем | |
| Уçлăхри йĕр | Винт йĕрĕ • Тăвайккилле йĕр • Локсодрома • Ортодроми • Губка |
| Конусла касăлусем | |
| 3-мĕш рет | Эллипсла:
Эллипсла йĕр •
Якоби функцийĕсем •
Интеграл •
Функцисем |
| Лемнискатсем | Бернулли (Кассини çаври) • Бута • Жероно |
| Аппроксимацилле | |
| Спиральсем | Архимедла (Фермалла) • Гиперболăлла • «Жезл» • Клотоида • Логарифмла |
| Циклоидăлласкерсем | Циклоида • Эпициклоида (Кардиоида • Нефроида) • Гипоциклоида (Дельтоида (Штейнер кукрашки) • Астроида) • Трохоида (Тăснăскер + Укороченная циклоида) • Эпитрохоида (Таснăскер + Кĕскетнĕ эпициклоида • Паскаль шуйĕ • «Кĕлчечек») • Гипотрохоида • Хăвăрт ану (Брахистохрона, циклоида пĕки) |
| Фракталлисем | Кох • Леви • Минковский • Пеано • Топологи (Салфетка + Серпинский кавирĕ • Менгер губки) |
| Урăххисем | Квадратриса • Погони (Трактриса) • Трохоида • Сăнчăрлă йĕр (пуçхĕрлĕ арка) • Безье • Пĕр тан анлă • Синусоида |